ABSTRACT: Three-dimensional finite-difference or finite-volume models of sinuous open channels (e.g., narrow rivers, estuaries, and reservoirs) generally require boundary-fitted grids and curvilinear flow solution. Cartesian models with square grid cells are simpler to apply, but require a larger number of cells, as the cell size is determined by cross-stream resolution. This paper presents a simplified curvilinear approach suitable for systems where the along-stream length scale is larger than the cross-stream scale. The curvilinear Navier-Stokes equations are manipulated so the left-hand side is identical to the Cartesian momentum equations. The right-hand side then consists of grid-stretching curvature terms. These terms are written as functions of a perturbation parameter, so the first-order curvilinear effects are obtained with the lowest-order perturbation terms. As the Cartesian equations’ form is preserved, we can readily adapt a Cartesian model to this perturbation curvilinear approach by adding the small curvilinear terms as explicit momentum sources.

EXTRACT: Figure 5:

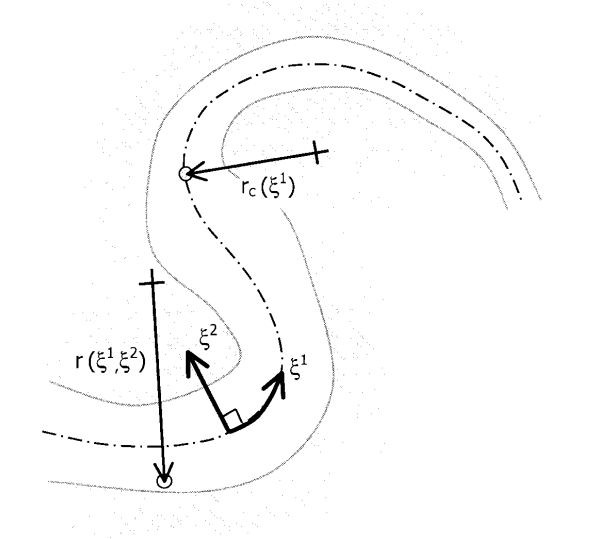
Fig. 5. Relationship between Centerline Radius of Curvature (r_c), Local Radius of Curvature (r) and Curvilinear Coordinate Direction \xi^2 and \xi^2